Shannon-Wiener index or Shannon index is an established measure of biodiversity in ecological studies. It is a measure of relative abundance of a set of organisms defined by,

where pi is the proportion of i th species in the community
ln indicates logarithm
Shannon index increases as the number of species classes increases, or the proportional distribution among species types become more equitable. For a given number of species classes, the maximum value of the Shannon index is reached when all classes have the same proportion. The logarithm is conventionally taken to the base two, but of late, natural logarithm is admitted.
This index, although efficient, has a significant deficiency. Suppose two plots have the same number of species with the same relative abundances (pi ). Shannon’s index will return the same value for H, indicating that the two plots are equally diverse. However, if the first plot contains species each from a different family and in the other plot, all the species are from the same family, the more diverse state of the first plot does not get reflected in the Shannon’s measure. Hence this index needs to be modified. Some proposals are made here, with illustrations. An extension of the Shannon index up to the family level is presented here.
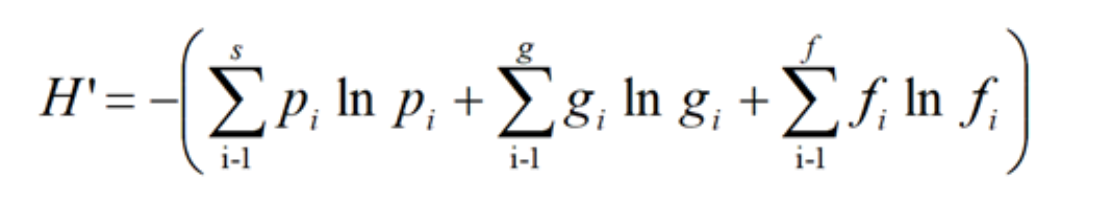
where pi is the proportion of i th species in the community
gi is the proportion of i th genus in the community
fi is the proportion of i th family in the community
s, g, f indicate the number of species, number genera, and number of families respectively
ln indicates logarithm
There is no problem with scaling here as all the components individually are based on proportions that add up to unity.
Equation (2) can more tersely be expressed as
H‘ = Hs + Hg + Hf
The situation is described using an artificial set of data for two plots (Table 1). The first site contains 12 species belonging to 6 genera and two families. The second site includes 12 species belonging to a single genus of a single family. The number of individuals 1under each group is taken as 10 for convenience. The different components of the modified index are presented in the last column.
In the first plot, the overall index is almost double that of the second plot because of the higher genus and family diversity. The basic measure of species diversity (Hs) remains the same for the two plots. It is important to consider this aspect of diversity in all future studies in ecology.
Status of two plots using artificial data:
| Site | Family | Genus | Species | Abun -dance |
Index |
|---|---|---|---|---|---|
| 1 | F1 | F1G1 | F1G1S1 | 10 | |
| 1 | F1 | F1G1 | F1G1S2 | 10 | |
| 1 | F1 | F1G2 | F1G2S1 | 10 | |
| 1 | F1 | F1G2 | F1G2S2 | 10 | |
| 1 | F1 | F1G3 | F1G3S1 | 10 | |
| 1 | F1 | F1G3 | F1G3S2 | 10 | |
| 1 | F2 | F2G1 | F2G1S1 | 10 | |
| 1 | F2 | F2G1 | F2G1S2 | 10 | |
| 1 | F2 | F2G2 | F2G2S1 | 10 | |
| 1 | F2 | F2G2 | F2G2S2 | 10 | Hs = 2.48 |
| 1 | F2 | F2G3 | F2G3S1 | 10 | Hg = 1.79 |
| 1 | F2 | F2G3 | F2G3S2 | 10 | Hf = 0.69 |
| H’ = 4.96 | |||||
| 2 | F1 | F1G1 | F1G1S1 | 10 | |
| 2 | F1 | F1G1 | F1G1S2 | 10 | |
| 2 | F1 | F1G1 | F1G1S3 | 10 | |
| 2 | F1 | F1G1 | F1G1S2 | 10 | |
| 2 | F1 | F1G1 | F1G1S4 | 10 | |
| 2 | F1 | F1G1 | F1G1S5 | 10 | |
| 2 | F1 | F1G1 | F1G1S6 | 10 | |
| 2 | F1 | F1G1 | F1G1S7 | 10 | |
| 2 | F1 | F1G1 | F1G1S8 | 10 | |
| 2 | F1 | F1G1 | F1G1S9 | 10 | |
| 2 | F1 | F1G1 | F1G1S10 | 10 | Hs = 2.48 |
| 2 | F1 | F1G1 | F1G1S11 | 10 | Hg = 0 |
| 2 | F1 | F1G1 | F1G1S12 | 10 | Hf = 0 |
| H’ = 2.48 |

